1.3 Persamaan Kuadratik


1.3 Persamaan Kuadratik

1.   Persamaan Kuadratik adalah persamaan yang memenuhi syarat-syarat berikut:
(a)  Ia mengandungi tatatanda kesamaan, ‘=’
(b)  Ia melibatkan hanya satu anu.
(c)  Kuasa tertinggi anu terlibat ialah 2.

Contoh,


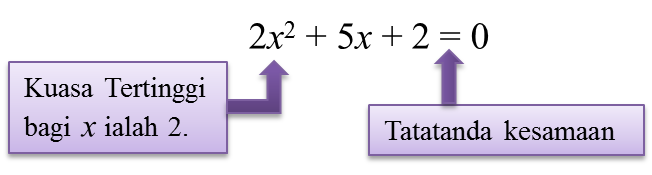
2.   Bentuk am bagi sesuatu persamaan kuadratik boleh ditulis sebagai:
 (a)  ax2 + bx + c = 0,
di mana a ≠ 0, b ≠ 0 dan c ≠ 0,
contoh: 4x2 + 13x – 12 = 0

   (b)  ax2 + bx = 0,
di mana a ≠ 0, b ≠ 0 tetapi c = 0,
contoh: 7x2 + 9x = 0

   (c)  ax2 + c = 0,
di mana a ≠ 0, c ≠ 0 tetapi b = 0,
contoh: 9x2 – 3 = 0


Contoh 1:
Tulis setiap persamaan kuadratik berikut dalam bentuk am.
(a)  x2– 5x = 12
(b)  –2 + 5x2 – 6x = 0
(c)  7p2 – 3p = 4p2 + 4p – 3
(d)  (x – 2)(x + 6) = 0
(e)  3 – 13x = 4 (x2 + 2)
( f ) 2 − y = 1 − 3 y y ( g ) p 4 = 2 p 2 − 3 10 ( h ) y 2 + 5 4 = y − 1 2 ( i ) 4 p 7 = p ( 7 p − 6 )


Penyelesaian:
Bentuk am bagi sesuatu persamaan kuadratik ialah ax2 + bx + c = 0
(a)  x2– 5x = 12
x2– 5x -12 = 0

(b)  –2 + 5x2 – 6x = 0
5x2 – 6x –2 = 0

(c)  7p2 – 3p = 4p2 + 4p – 3
7p2 – 3p – 4p2 – 4p + 3 = 0
3p2 – 7p  + 3 = 0

(d)  (x – 2)(x + 6) = 0
x2+ 6x – 2x – 12 = 0
x2+ 4x – 12 = 0


(e)  3 – 13x = 4 (x2 + 2)
3 – 13x = 4x2 + 8
–4x2 – 8 + 3 – 13x = 0
–4x2 – 13x – 5 = 0
4x2 + 13x + 5 = 0

( f ) 2 − y = 1 − 3 y y
2y – y2 = 1 – 3y
2y – y2 – 1 + 3y = 0
 – y2 + 5y – 1 = 0
y2– 5y + 1 = 0

  ( g ) p 4 = 2 p 2 − 3 10
10p = 8p2 – 12
–8p2 + 10p +12 = 0
8p2 – 10p – 12 = 0

( h ) y 2 + 5 4 = y − 1 2
2y2 + 10 = 4y – 4
2y2 – 4y + 10 + 4 = 0
2y2 – 4y + 14 = 0

( i ) 4 p 7 = p ( 7 p − 6 )
4p = 7p (7p – 6)
4p = 49p2 – 42p
– 49p2 + 42p + 4p  = 0
49p2 – 46p = 0


Leave a Comment